Request Demo
Last update 29 Aug 2025

University of Dschang
Last update 29 Aug 2025
Overview
Corporation Tree
Boost your research with our corporation tree data.
login
or

Pipeline
Pipeline Snapshot as of 11 Oct 2025
No data posted
Login to keep update
Deal
Boost your decision using our deal data.
login
or

Translational Medicine
Boost your research with our translational medicine data.
login
or

Profit
Explore the financial positions of over 360K organizations with Synapse.
login
or

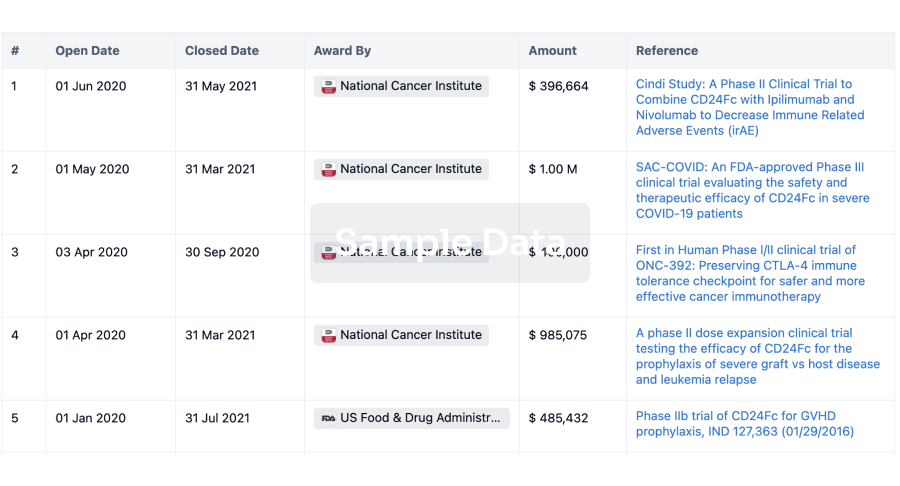
Grant & Funding(NIH)
Access more than 2 million grant and funding information to elevate your research journey.
login
or
Investment
Gain insights on the latest company investments from start-ups to established corporations.
login
or
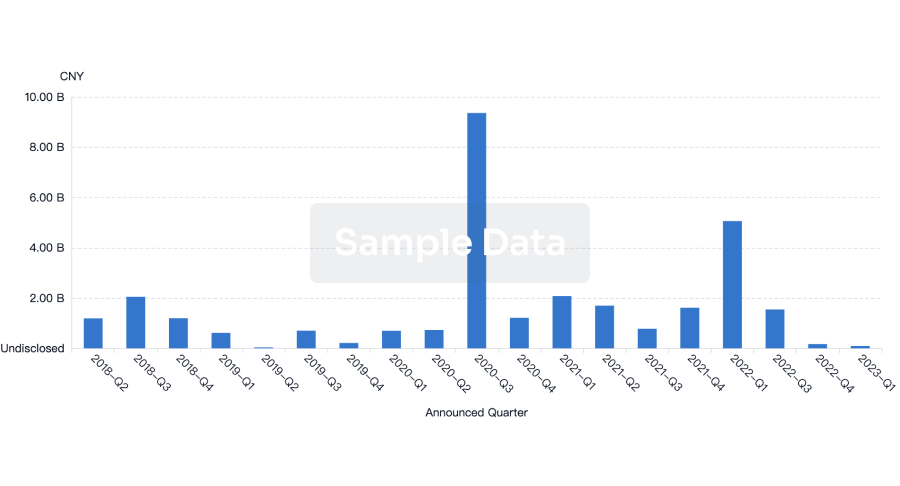
Financing
Unearth financing trends to validate and advance investment opportunities.
login
or

AI Agents Built for Biopharma Breakthroughs
Accelerate discovery. Empower decisions. Transform outcomes.
Get started for free today!
Accelerate Strategic R&D decision making with Synapse, PatSnap’s AI-powered Connected Innovation Intelligence Platform Built for Life Sciences Professionals.
Start your data trial now!
Synapse data is also accessible to external entities via APIs or data packages. Empower better decisions with the latest in pharmaceutical intelligence.
Bio
Bio Sequences Search & Analysis
Sign up for free
Chemical
Chemical Structures Search & Analysis
Sign up for free
